Well, well, well.
My question about optimal locations to hold a party turns out to have been harder than I expected. As always, my super-intelligent commenters made some good observations (read the comments on the other thread).
Taking the biscuit, however, was my colleague Lee Hollingdale. He decided that, whilst theory is nice, nothing beats a good bit of empiricism: he knocked up a program so that I could experiment for real. Wow.
Here are the results of Lee's program.
Firstly, let's look at the "two friend" case. The brighter dots represent points of minimum "total distance travelled". As we see, our prediction that it would be the entire line between the two friends was correct:
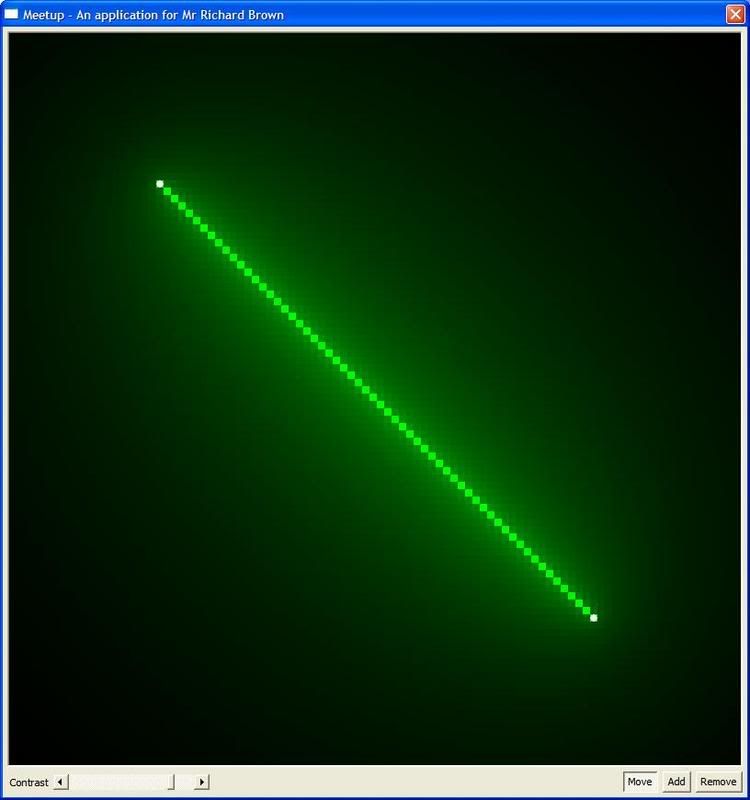
Now, if we add an extra point - along the same line, something interesting happens. The optimal position is now just that single new point:

If, instead, we move the third friend to somewhere not on the line, but still close, it seems that their house is the best place to hold the party:

But if they move out into zone 6, it rapidly becomes clear that their house is not appropriate (quite right, too).
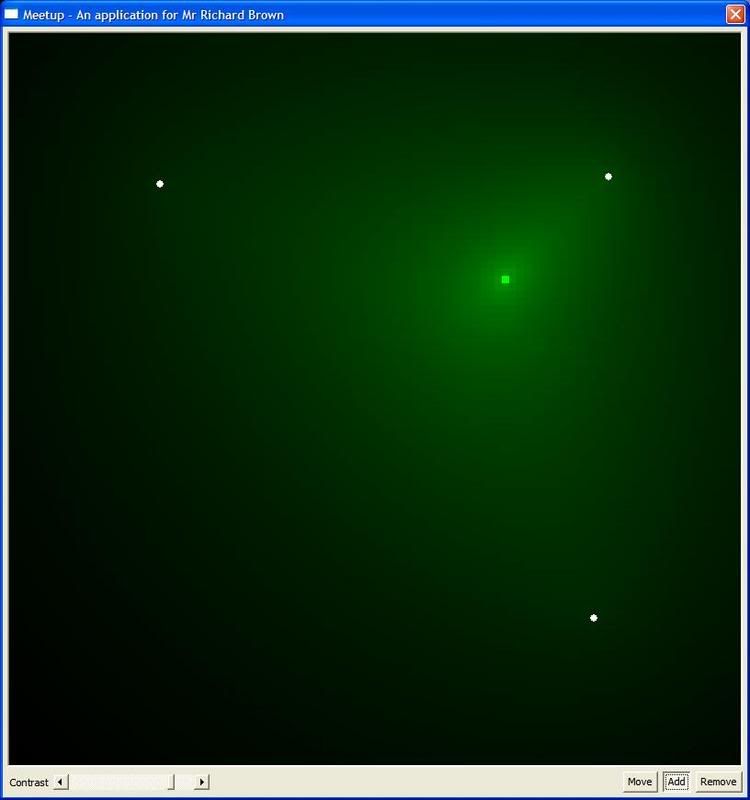
And now, if we pretend to have loads of friends, the optimal point (and there is just one point) can be seen:

So, it does appear that two friends is the special case but the "hold the party at the third friend's house" result is not one I was expecting
Thanks Lee!

7 comments:
Good grief.
"if we pretend to have loads of friends"
That's what Twitter is for, right? :-)
Lovely app.
In the one where the middle point is slightly off the line, the best place to have the party isn't actually at the middle point, it's just very close to it.
Glad you liked the app. I need to get out more, apparently.
Have you tried the even-number-of-people-on-a-straight-line case? One of your original commenters mentioned this, and the app shows it nicely...
@lee: re: multiple points on a straight line... very nice :-) How cool is that?!
@roo: yes. Thank you for that :-p
@kyb: good spot. (perhaps we should hold it in their garden :-) )
Interesting, I like the app and the details.
Just a thought here...
Taking the friends meeting scenario, in reality, unless you are on a large field or in the desert, you would have to use streets and maybe some means of transportation. So I think the problem is actually a graph problem rather than a geometry problem.
Hi Peter,
Good point. We did make the simplifying assumption of no buildings and no hills. Even so, it was a poor approximation.
Perhaps a graph would have been better.
Grrr.
Back to the drawing board.
Post a Comment